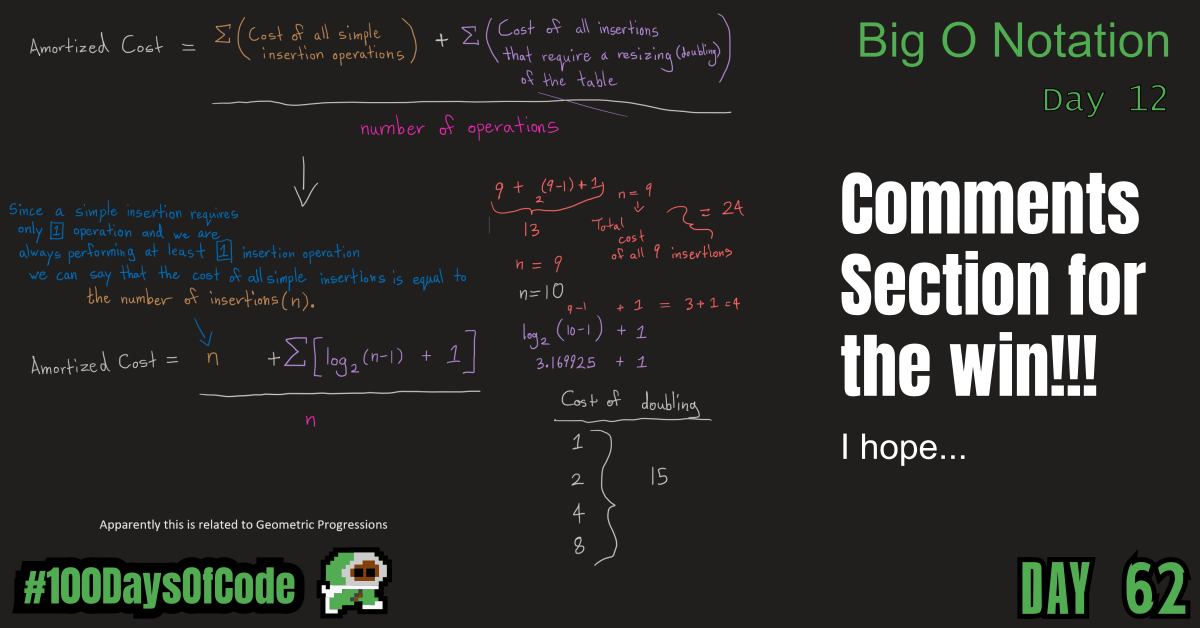
A little bit more progress today. I got back to the writing manually to help me make sense of what I have been missing in the aggregate method. I think I finally understand everything up until the final math representation. Based on a few comments I finally know where to look. Apparently the step that has been missing lies in Geometric Progressions. I am still a little lost so hopefully this will work out. Man this has taken me a long time to understand.

TLDR;

Okay, so here are the highlights of what I did:

• I am still trying to breakdown the Aggregate Method. I found the comments for this article from Geeks for Geeks to be very helpful. I thankfully have a direction. The combo of rewriting every step involved in the calculation by hand alongside reading the comments has helped me feel like I can actually understand this. I have never been more grateful for a comment section than I have been today.
• I started looking into Geometric progressions. I am not familiar with the term but I hope it’s not too difficult to learn. The concept is in math and presents a potential way to solve for x. At least that’s what the example solution used to solve for the O(n) of the example problem for the Aggregate Method.

Conclusion

That’s all for today. If you are interested in the MIT course you can check out the video lecture I’m currently going through. The lecture is helpful but isn’t sufficient by itself. Anyways, until next time PEACE!